This blog details a non-exhaustive list of ideas I believe are important in raising attainment in mathematics. All of these are part of my own planning as a head of department.

1. Culture

Teacher Culture: It is fine to be skeptical, but less so to be cynical. (There are obvious exceptions, of course!) We need to give new ideas and approaches a chance. We need to be willing to embrace and try things out. We need to believe that what we do can have a positive impact upon the attainment and, more importantly, the lives of our pupils. If we do not believe that what we do is purposeful, then why be a teacher? If we do not believe that we can make a positive difference then we might as well go off and do something else. Professional development, reading and discussion about learning and teaching should be central to a department. In John Hattie’s, often criticised, meta-analyses two of the top ranking effects come from: a teacher’s estimates of pupil achievement (the danger of a negative self fulfilling prophecy) and collective teacher efficacy (how we work together collectively to improve). (1), (2)

Pupil Culture: We need to establish the conditions where pupils see that they are progressing in their mathematics and that they can feel the sense of satisfaction that comes with improvement. We need to ensure that we have high expectations of all pupils while ensuring that our approaches are equitable and extra provision is directed at those who require it. The single most powerful way of improving pupil motivation is by increasing prior attainment. Garon Carrier et al. (3) demonstrated that “achievement level in mathematics was fairly well established early in primary school, and subsequently predicts intrinsic motivation toward mathematics.” Motivation did not, however, predict achievement. Greg Ahsman (4) shared the below graphic.

2. Quality of Curriculum Enactment

Teachers in some jurisdictions have an advantage over teachers here in Scotland. The quality of Initial Teacher Education (ITE) and ongoing professional development is rooted in the subject rather than in general principles. The curricula in these countries is well planned and exemplified. We do not have this in Scotland.

In South Korea,(5) for example. the curriculum details:

• objectives (why to teach/learn)
• content (what to teach/learn)
• progression (when to teach/learn)
• instruction (how to teach/learn).

This would be incredibly useful. Instead we have a situation where the knowledge required to deliver the curriculum meaningfully isn’t written down for teachers. Instead, we have broad headlines – a wish list of statements such as “children will be able to… having experienced….”. It is much harder for teachers in Scotland to enact the curriculum than it is for teachers in South Korea. We know what content is to be covered, but nobody seems to have done the deep thinking about how things can and should connect together.

Martin Simon (6) described the idea of learning trajectories. These have three components:

1. A set of mathematical goals.
2. A clearly marked developmental path.
3. A coherent set of instructional tasks or activities.

These are not present in the Scottish curriculum, so what can we do instead? Sadly, the answer seems to be trial and error, or in a phrase which sounds less amateur – develop and iterate. We have to develop learning trajectories of our own. We might depend upon textbooks – but these are limited. There are no textbook series in Scotland, for secondary, which are constantly excellent. Many have positive features, but most exhibit one or several issues: repetitive and procedural tasks only, no utilisation of visual models, little focus on conceptual understanding, scarcity of problem solving questions, too few or too many questions on a topic etc etc.

It is because of this that I have, along with Siobhan McKenna and Gary Lamb, began to develop our own curriculum implementation centred around our own booklets. These try to address the concerns I’ve raised above. When I spoke to Tom Francome when writing my book he used the phrase ” a cannon of tasks” which all pupils should get to experience. I like this and think that

3. Consistency of Models and Metaphors

Closely related to the curriculum, but worth mentioning on its own. We need to ensure that as part of our progression planning we are coherent in the models that we utilise. For instance the grid model for multiplication lends itself to long division, distributive law in algebra, factorisation, completing the square and algebraic long division. This seems like a model worth pursuing. Similarly the metaphor of “zero pairs” using algebra discs/tiles can be extended into other ideas later in the curriculum. It is important to consider what strands we might want to develop through the curriculum. In Japan, for instance, the double number line plays a key role throughout. The relationships between values on either line demonstrate proportion. For instance:

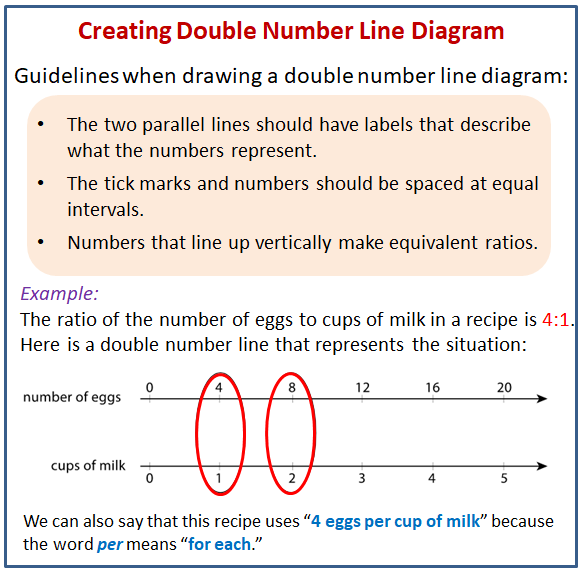
Eventually, the the line representing the independent variable is rotated 90^o to the left and we end up with the x and y axis. A seamless transition to linear relationships. This is the power of having joined up thinking. This is not to say that we should stick with only a couple of representations. In my experience utilising multiple representations of an idea can be useful. It can be useful to come at an idea in several different ways. Consider the following diagrams which Siobhan created for one of our booklets.

The subtle shift in how the model is used helps to convey an alternative, but related meaning.

Overall, a small number of representations at the core, which permeate the curriculum, that pupils can become comfortable with, which we return to, time and again, is incredibly powerful (and perhaps time saving, in the long term).

4. The diet of tasks

Tasks play a central role in mathematics classrooms. I have written a whole book about how important they are. Lappan and Briars argue that ‘there is no decision that teachers make that has a greater impact on pupils’ opportunities to learn and on their perceptions about what mathematics is than the selection or creation of the tasks.’ (Lappan and Briars) (7)

Essentially, it’s not just about how we teach, it’s about what we ask pupils to do and to think about, regarding what we are teaching. If pupils only ever do procedural tasks then we would be naive to expect the development of conceptual understanding of of competence in problem solving. It is through a rich diet of mathematical tasks that we provide the space for pupils to engage in varying types of mathematical activity. You can see throughout this website and in the curriculum booklets a variety of some tasks types. If curriculum is about macro-design then tasks are micro-design. How we join up tasks into coherent sequences is the reality of the lived experience of the curriculum for our pupils.

Finally, we need to ensure that the tasks we ask pupils to engage in utilise the models and metaphors that we have decided as being central to our curriculum journey. For instance, many of my negative number tasks use the models I want teachers to utilise in class.

5. Pedagogical approaches – adaptable

As I wrote in my book: “There is no holy grail of teaching, there is simply what people are able to do, based on their past experiences. Pedagogy is deeply individual: it is a result of our experiences, our understanding of mathematics and our beliefs around how to teach it. It might be influenced by the external examinations which hold the system to account. At its core, pedagogy is rooted in the relationships we make with the pupils in our classes. We may employ different pedagogies with different groups. Even with the same group, our approach might change depending on the circumstances.”

It is imperative that we do not fall into the trap of believing teaching is a dichotomy of progressive versus traditional. Explicit instruction is powerful. There is a great deal of professional knowledge about different techniques related to this. Example-problem pairs with backwards fading is one such example. However, there is more to teaching than clear explanations and telling. The space for pupils to discuss and explain their ideas is vital. How are we to develop the mathematical sense of an individual if their teacher is the one who asks all of the questions and solves all of the problems? There is over a century of literature on effective mathematics teaching. The the use of concrete materials and visual models is well documented, for instance. These allow us to make better inferences about pupils understanding while providing space for the making of meaning and connections. It is difficult to visualise the understanding of another person; using different representations allows teacher and pupils to build a shared ‘concept image’ on which to base discussions. 

Malcolm Swan (8) argued that ‘traditional, “transmission” methods in which explanations, examples and exercises dominate do not promote robust, transferrable learning that endures over time or that may be used in non-routine situations.’ The key here is the word ‘dominate’. There is a place for explanations, examples and exercises – but they are inadequate on their own. On any given day, in any given lesson I imagine most of us are not entrenched in the transmission or collaborative camps. It depends.

My point is simple: a strong base of explicit teaching, with a variety of other pedagogical approaches which encourage collaboration, discussion and problem solving is possibly the “sweet spot”.

6. The mastery cycle

Mastery learning is a simple but robust formative assessment cycle.  Few, nowadays, would doubt the effectiveness of formative assessment and responsive teaching. Mastery learning is the best implementation of this. It can result in significant increases in attainment without any other change in instructional practice. Professor Thomas Guskey recently said in an interview “There is probably no other innovation or strategy which has been thoroughly investigated and been consistently found to be effective than mastery learning.”

I have previously written about this a lot, including a deep dive blog, where you can read more about what is involved. A great read on the idea is this from Thomas Guskey.

Another key message to arise in the evaluation studies is that teachers perceptions of what their pupils can achieve increase in mastery learning classrooms, due to the effectiveness of the approach itself. This helps us to address the idea of professional culture I raise in point 1.

Conclusions

This blog has outlined a few “big ideas” for consideration in attempting to raise attainment in mathematics. In part 2 (whenever I get round to it) I will outline some thoughts around memory, homework, interventions and setting versus mixed attainment.

As always, comments and questions welcome.

References

(1) Mucella Uluga*, Melis Seray Ozdenb, Ahu Eryilmazc (2011). The effects of teachers’ attitudes on students’ personality and performance.

(2) Hattie, John (2017). Visible Learning https://visible-learning.org/backup-hattie-ranking-256-effects-2017/

(3) Garon Carrier et al (2015), Intrinsic motivation and achievement in mathematics in elementary school: A longitudinal investigation of their association https://www.researchgate.net/publication/282135309_Intrinsic_motivation_and_achievement_in_mathematics_in_elementary_school_A_longitudinal_investigation_of_their_association

(4) Ashman (2015) Motivating students about maths https://gregashman.wordpress.com/2015/11/12/motivating-students-about-maths/

(5) Ferreras, A., Kessel, C. and Kim, M. (2015) Mathematics curriculum in Korea. In Mathematics Curriculum, Teacher Professionalism, and Supporting Policies in Korea and the United States. Summary of a Workshop. Washington, DC: National Academies Press, pp. 9–22.

(6) Simon, M. A. (1995) Reconstructing mathematics pedagogy from a constructivist perspective. Journal for Research in Mathematics Education 26 (2) pp. 114–145.

(7) Lappan, G. and Briars, D. (1995) How should mathematics be taught? In Carl, I. M. (ed.) Seventy-five years of progress: prospects for school mathematics. Reston, Virginia: National Council of Teachers of Mathematics, pp. 115–156.

(8) Swan, M. (2006) Collaborative learning in mathematics. Leicester: National Institute of Adult Continuing Education. http://twittermathcamp.pbworks.com/w/file/fetch/98345576/Collaborative%20Learning%20in%20Mathematics.pdf